Playing with Probability - Efrons Dice: Difference between revisions
SimonKnight (talk | contribs) No edit summary |
No edit summary |
||
| (21 intermediate revisions by 4 users not shown) | |||
| Line 1: | Line 1: | ||
[[Category:Maths]][[Category:Secondary]][[Category:ORBIT]] | [[Category:Maths]][[Category:Secondary]][[Category:ORBIT]] | ||
{{DISPLAYTITLE:Playing with Probability - Efron's Dice}} | |||
{{Rinfo | {{Rinfo | ||
|type= Lesson idea | |||
|attribution={{MarkDawes}} | |||
|title=Playing with Probability - Efron's Dice | |title=Playing with Probability - Efron's Dice | ||
| | |tagline=I have some dice that are coloured green, yellow, red and purple... | ||
|image=Nets.png | |||
|topic=Probability | |||
|subject=Maths | |subject=Maths | ||
|resourcenumber= M0021 | |resourcenumber= M0021 | ||
|age= | |age= KS4, KS3, Secondary | ||
|content= A lesson activity to explore probability | |content= A lesson activity to explore probability with dice. We are used to the idea of transitivity, where we can ascribe an order to events. Efron’s dice are non-transitive and probability methods that the pupils are familiar with can be used to explore how to play a game using them. | ||
|strategy= | |strategy= | ||
|additional resources= | |additional resources= | ||
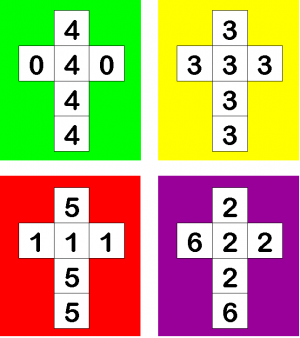
|useful information= | |useful information= Large wooden dice that are coloured green, yellow, red and purple with stickers to show numbers (see [[Playing with Probability - Efrons Dice/Activity|this page]]). <br> Any practical probability starting point carries the risk that the results will not, in the short term, produce the expected results. This is a useful discussion point. | ||
|Learning Objectives= | |Learning Objectives= Understanding the worth of probability tables and how to use them to solve a problem. | ||
|related resources= | |related resources= | ||
|other= | |other= | ||
|format= wiki page | |final=yes | ||
|resources= [[Efron's Dice Activity]] | |licence= | ||
|format= A wiki page also available as a downloadable Word document. | |||
|resources= [[Playing with Probability - Efrons Dice/Activity]] and as a download [[file:Efron's Dice Activity.doc]]. | |||
}} | }} | ||
Latest revision as of 11:56, 17 November 2012
Lesson idea. A lesson activity to explore probability with dice. We are used to the idea of transitivity, where we can ascribe an order to events. Efron’s dice are non-transitive and probability methods that the pupils are familiar with can be used to explore how to play a game using them.
Teaching approach. Efron's dice provide a discussion(ta) topic for joint reasoning(ta) - whole class(ta) or in group work(ta). Pupils can explore aspects of mathematical thinking(ta) particularly with relation to probability. (edit)
| Resource details | |
| Title | Playing with Probability - Efron's Dice |
| Topic | [[Topics/Probability|Probability]] |
| Teaching approach | [[Teaching Approaches/Whole class|Whole class]], [[Teaching Approaches/Mathematical thinking|Mathematical thinking]], [[Teaching Approaches/Group work|Group work]], [[Teaching Approaches/Reasoning|Reasoning]], [[Teaching Approaches/Discussion|Discussion]] |
| Learning Objectives | Understanding the worth of probability tables and how to use them to solve a problem. |
| Format / structure | A wiki page also available as a downloadable Word document. |
| Subject | [[Resources/Maths|Maths]] |
| Age of students / grade | [[Resources/Secondary|Secondary]], [[Resources/KS4|KS4]], [[Resources/KS3|KS3]]
|
| Useful information | Large wooden dice that are coloured green, yellow, red and purple with stickers to show numbers (see this page).
|
| Files and resources to view and download | Playing with Probability - Efrons Dice/Activity and as a download File:Efron's Dice Activity.doc. |
| Acknowledgement | This resource was adapted from resources contributed by Mark Dawes |