Pick your resource by topic:

- [[Topics/Algebra|Algebra]]
- [[Topics/Assessment|Assessment]]
- [[Topics/Blogs|Blogs]]
- [[Topics/Blogs|Blogs]]
- [[Topics/E-skills|E-skills]]
- [[Topics/Copyright|Copyright]]
- [[Topics/Consecutive Sums|Consecutive Sums]]
- [[Topics/E-skills|E-skills]]
- [[Topics/E-safety|E-safety]]
- [[Topics/Sharing practice|Sharing practice]]
- [[Topics/Curriculum development|Curriculum development]]
- [[Topics/Virtual Learning Environment|Virtual Learning Environment]]
- [[Topics/E-skills|E-skills]]
- [[Topics/Digital Media|Digital Media]]
- [[Topics/ICT|ICT]]
- [[Topics/Wikis|Wikis]]
- [[Topics/Multimedia|Multimedia]]
- [[Topics/ICT|ICT]]
- [[Topics/Games|Games]]
- [[Topics/Handling Data|Handling Data]]
- [[Topics/ICT|ICT]]
- [[Topics/Investigation|Investigation]]
- [[Topics/Modelling|Modelling]]
- [[Topics/Models|Models]]
- [[Topics/Multimedia|Multimedia]]
- [[Topics/Probability|Probability]]
- [[Topics/Scientific literacy|Scientific literacy]]
- [[Topics/Science citizenship|Science citizenship]]
- [[Topics/Reading skills|Reading skills]]
- [[Topics/Simultaneous Equations|Simultaneous Equations]]
- [[Topics/Standard Index Form|Standard Index Form]]
- [[Topics/Statistics|Statistics]]
- [[Topics/Wikis|Wikis]]
- [[Topics/Writing|Writing]]

Relevant resources

Algebra	What's Possible?
	Many numbers can be expressed as the difference of two perfect squares. What do you notice about the numbers you CANNOT make? This lesson idea is about exploring and noticing structure^(ta). The collection of NRICH activities are designed to develop students capacity to work as a mathematician. Exploring, questioning, working systematically, visualising, conjecturing, explaining, generalising, justifying, proving are all at the heart of mathematical thinking. This particular resource has been adapted from an original NRICH resource. NRICH promotes the learning of mathematics through problem solving. NRICH provides engaging problems, linked to the curriculum, with support for teachers in the classroom. Working on these problems will introduce students to key mathematical process skills. They offer students an opportunity to learn by exploring, noticing structure and discussing their insights, which in turn can lead to conjecturing, explaining, generalising, convincing and proof. The Teachers’ Notes provided focus on the pedagogical implications of teaching a curriculum that aims to provoke mathematical thinking. They assume that teachers will aim to do for students only what they cannot yet do for themselves. As a teacher, consider how this particular lesson idea can provoke mathematical thinking. How can you support students' exploration? How can you support conjecturing, explaining, generalising, convincing and proof?.
Assessment	Using Assessment to Raise Achievement in Maths
	Learning goals; self & peer assessment; effecting questioning; marking and case studies This resource explores approaches to assessment^(ta) in maths, including the sharing of learning objectives^(ta), group work^(ta), whole class^(ta) assessment, questioning^(ta) and more. Four case studies serve as useful discussion prompts to share practice^(ta). This .doc version of the QCA's 'Using assessment^(ta) to Raise Achievement in Maths' allows schools to select parts of the document that are most relevant to them.
Assessment	Changing KS3 Questions for Engaging Assessment
	A large set of questions grouped by topic, paper, and national curriculum level Test questions are often seen as uninteresting and useful only to assess pupils summatively. This resource however allows questioning^(ta) to be used to support pupils’ revision, creativity and higher order^(ta) problem-solving in class. The tasks could be conducted via whole class^(ta) discussion^(ta) or assessment^(ta), perhaps using mini-whiteboards^(tool), or in small group work^(ta) situations.
Blogs	Getting a buzz out of blogging
	Starting with the basic principles of blogs^(tool) and blogging, this primary course looks at their use within education and how they can be used to improve teaching and learning. The focus is particularly on blogs as an ICT⁽ⁱ⁾ tool for collaboration^(ta) which encourages the effective use of reasoning^(ta) and language^(ta). The unit also discusses practical elements such as e-skills^(topic) and copyright^(topic) issues you might encounter in blogging.
Consecutive Sums	Using Prime and Square Numbers - How Old Am I?
	Last year I was square, but this year I am in my prime. How old am I? This short activity offers opportunity for pupils to engage in mathematical thinking^(ta) and higher order^(ta) problem solving/reasoning^(ta). They should be able to make links between different areas of mathematics and explore their ideas in whole class^(ta) discussion^(ta) and questioning^(ta).
Digital Media	21st century show and tell
	A DEFT case study with Dinnington Comprehensive, Rotherham This cross curricula⁽ⁱ⁾ case study focusses on Digital Literacy, in particular using E-skills⁽ⁱ⁾ to: support skills in writing/recording for a target audience and to improve communication and research skills through the process of creating OER⁽ⁱ⁾s. The case study illustrates issues involved in the use of video⁽ⁱ⁾ for educational purposes, with an emphasis on students producing and releasing OERs. The method could also be used for self/peer assessment⁽ⁱ⁾ with pupils. One of the lesson ideas from the case study is available as a separate resource at Creating Instructional Videos.
Games	Introduction to games
	This courses provides an introduction to games^(tool) in the classroom. The course will focus on freely available online games, which can provide a starting point for exploring their use in the classroom without investment in hardware and software. At the conclusion of this course you will have engaged in lesson planning^(ta), ready for implementation in your classroom.
Handling Data	Olympic Records
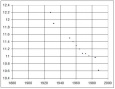
	Can you deduce which Olympic athletics events are represented by the graphs? This lesson idea is about reasoning, justifying, convincing and proof^(ta). The collection of NRICH activities are designed to develop students capacity to work as a mathematician. Exploring, questioning, working systematically, visualising, conjecturing, explaining, generalising, justifying, proving are all at the heart of mathematical thinking. This particular resource has been adapted from an original NRICH resource. NRICH promotes the learning of mathematics through problem solving. NRICH provides engaging problems, linked to the curriculum, with support for teachers in the classroom. Working on these problems will introduce students to key mathematical process skills. They offer students an opportunity to learn by exploring, noticing structure and discussing their insights, which in turn can lead to conjecturing, explaining, generalising, convincing and proof. The Teachers’ Notes provided focus on the pedagogical implications of teaching a curriculum that aims to provoke mathematical thinking. They assume that teachers will aim to do for students only what they cannot yet do for themselves. As a teacher, consider how this particular lesson idea can provoke mathematical thinking. How can you support students' exploration? How can you support conjecturing, explaining, generalising, convincing and proof?.
ICT	Data Logging and Control
	A compendium with numerous ideas for using sensors to teach science. This book provides a set of resources and lesson ideas with ICT⁽ⁱ⁾ as a key focus for use in inquiry^(ta) based learning and the scientific method^(ta). It offers opportunities for use of group work^(ta) and collaboration^(ta) as well as whole class^(ta) questioning^(ta).
ICT	Creating Instructional Videos
	Children create instructional videos to upload to YouTube This activity is a cross-curricular^(subject) activity with a literacy focus, involving a collaborative^(tool) approach, giving children to opportunity to work together to produce a set of instructional resources. Children were encouraged to engage in group talk^(ta) and discussion^(ta) in the classroom to reflect on what they should include in their videos. The activity furthers e-skills^(topic) through the use of whole class^(ta) participation. It develops e-safety^(topic) skills through discussion of the issues relating to posting digital content online. Children were allowed to choose their own subject for the video, although this could be set by a teacher with a specific outcome in mind, or could be tailored to cover a particular topic or subject. It could, for instance, be used to explain their mathematical thinking^(ta).
Investigation	Consecutive Sums
	Can all numbers be made in this way? For example 9=2+3+4, 11=5+6, 12=3+4+5, 20=2+3+4+5+6 By definition, a problem is something that you do not immediately know how to solve, so learning how to solve something unfamiliar is not straightforward. Tackling an extended problem is difficult. This lesson gives pupils an opportunity to engage in mathematical thinking^(ta) and develop their higher order^(ta) thinking skills on a problem that is accessible but which has interest. For example, the problem is presented in diagrammatic and numerical ways. The plan suggests several visualisation^(ta) methods to present the same underlying task. It should be useful for teachers to compare these different presentations and either to select the one that they feel will be most useful for their pupils or explore ways for the pupils to see the links between the different methods. The assessment^(ta) ideas, using other pupils' solutions from the NRICH website are widely applicable to other problems too.
Modelling	Models in Science
	Teachers use models to help pupils make sense of their observations An opportunity for teachers to discuss the use of modelling^(ta) and visualisation^(ta) in Key stage 3 science
Models	Different types of model in science
	"When you use a model, you should make it clear to pupils that it is a model that you are using." This resource provides some examples of the types of models^(tool) used in science teaching, their roles, and importance for teaching.
Multimedia	Working with multimedia
	In this unit, learners develop an understanding of contemporary digital media and how it applies to communication and entertainment ICT⁽ⁱ⁾s. This secondary course will show teachers how to use the e-skills^(topic) teaching and learning resources for this course to deliver the module in 60 teaching hours.
Probability	Playing with Probability - Efron's Dice
	I have some dice that are coloured green, yellow, red and purple... Efron's dice provide a discussion^(ta) topic for joint reasoning^(ta) - whole class^(ta) or in group work^(ta). Pupils can explore aspects of mathematical thinking^(ta) particularly with relation to probability.
Reading skills	Reading and discussing popular science articles
	Read. Get the world's view and see how science works for real The resource relates to the importance of: Scientific literacy^(topic). Science citizenship^(topic) - understanding science in its context. Literacy - understanding the role of different media in dissemination. Scientific understanding of particular concepts chosen. scientific language^(ta). scientific method^(ta). It can be delivered through a combination of homework^(ta) (perhaps to find an interesting article), group work^(ta) to explore various articles (perhaps in a carousel), and/or use of ICT⁽ⁱ⁾ including PowerPoint files to encourage students to present an area they are interested in.
Simultaneous Equations	Love Food, Hate Waste - Simultaneous Equations
	Using real world data to explore simultaneous equations Using a source that was not intended by its creators as a mathematical resource, pupils are introduced to informal ways of solving simultaneous equations. The lesson starts with an intriguing ‘hook’, pupils are able to use reasoning^(ta) skills to find an answer to the problem and can then, later, formalise this in an algebraic context, using their informal work to support the transition to mathematical thinking^(ta). whole class^(ta) work supports this inquiry^(ta) into the data provided. Using a resource not targeted at mathematics specifically encourages pupils to think about maths outside of the classroom.
Standard Index Form	An Introduction to the Standard Index Form
	Working out the rules according to which a calculator displays large numbers The Standard Index Form is a key idea for mathematicians and scientists. The notion that we choose to write numbers in this way requires some explanation. So in this activity, pupils take part in an investigation^(ta) on how standard index form works. This is a higher order^(ta) problem solving context where students are encouraged to engage in mathematical thinking^(ta). They may be involved in whole class^(ta) or small group work^(ta) discussion^(ta), so they have a good opportunity to practice using mathematical language^(ta) and questioning^(ta). This means that students do not need to be able to explain their ideas in full: they can use the calculator's feedback to discover whether their ideas are correct or not. This is also an exciting way for pupils to realise an initial idea that fits the data may need to be extended when new data arises. This resource therefore aims to develop investigative skills, as well as introduce pupils to standard index form in a memorable way. The pupils can later use their knowledge of indices in discussion^(ta) and group talk^(ta) as they explain what is happening.
Statistics	Cubic Equations and Their Roots
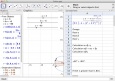
	To interactiviley explore and understand complex mathematics with GeoGebra This lesson features a ‘real life’ example for students to explore using visualisation^(ta) via GeoGebra. The focus on ‘real life’ increases student motivation. The activity engages pupils in group talk^(ta), mathematical thinking^(ta) and vocabulary^(ta). This open ended^(ta) task encourages higher order^(ta) thinking, and encourages whole class^(ta) discussion^(ta)/questioning^(ta) and inquiry^(ta) projects.